LONG PUT BUTTERFLY
| Strategy Details | |
| Strategy Type | Neutral, but bias can tilt slightly depending on the positioning of the middle strike at initiation |
| # of legs | 4 (Long 1 Lower Strike Put + Short 2 Middle Strike Puts + Long 1 Higher Strike Put) |
| Maximum Reward | Middle Strike Price - Lower Strike Price - Net Premium Paid |
| Maximum Risk | Limited to the extent of net premium paid |
| Lower Breakeven Price | Lower Strike Price + Net Premium Paid |
| Upper Breakeven Price | Higher Strike Price - Net Premium Paid |
| Payoff Calculation | Payoff of lower strike Long Put+ (2 * Payoff of middle strike Short Put) + Payoff of higher strike Long Put |
Explaination of the Strategy
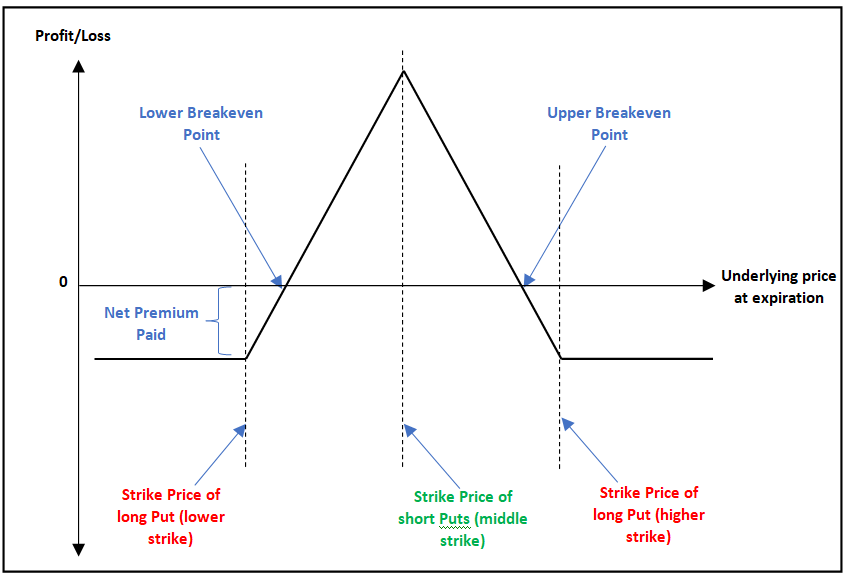
A Long Put Butterfly is a strategy that involves buying one lower strike Put, selling two middle strike Puts having the same strike, and again buying one higher strike Put. Each of these options must belong to the same underlying and must have the same expiration. The lower strike Put this is purchased is an OTM Put, the middle strike Puts that are sold are usually ATM Puts, and the higher strike Put this is purchased is an ITM Put. While the middle strike Puts that are sold are usually ATM, the trader could also initiate them as slightly ITM or slightly OTM. Doing so would, however, give a directional bias to the strategy. The default strategy that involves writing two ATM Puts is a neutral strategy. However, if the two Puts that are written are slightly ITM, the strategy becomes slightly bullish; and if the two Puts that are written are slightly OTM, the strategy becomes slightly bearish.
When executing this strategy, the trader must ensure that the lower and the higher strike that he/she chooses be equidistant from the middle strike. In other words, the price differential between the lower and the middle strike must equal the price differential between the middle and the higher strike.
This strategy has two breakeven points: lower and upper. As long as the underlying price is within the two breakeven points, the position is profitable. Under this strategy, maximum profit is achieved when the underlying price is exactly at the middle strike. As the Puts written at the time of initiation are ATM, the trader would want the underlying price to consolidate as much as possible at the middle strike to earn maximum possible profit under this strategy. Hence, time decay acts as a friend to the trader. On the other hand, if the underlying price either falls below the lower breakeven point or rises above the upper breakeven point, the trader would start suffering losses. Maximum loss under this strategy is limited to the extent of net premium paid and occurs when the underlying price falls below the lower strike or rises above the higher strike.
Because this strategy works best when the underlying price consolidates at or near the middle strike, this strategy benefits from low volatility. This is because the lower the volatility, the lower would be probability of the underlying price moving into the unprofitable zone. Hence, when entering into this strategy, ensure that you have a neutral view on the underlying and a bearish view on volatility.
Benefits of the Strategy
-
This strategy is a low cost strategy as the cost of the Puts bought is offset to a great extent by the two middle strike Puts sold
-
Maximum loss under this strategy is limited no matter how higher or lower the price of the underlying goes
-
The risk to reward ratio under this strategy can be quite attractive
Drawbacks of the Strategy
-
An unexpected rise in volatility can hurt the profitability of the trade, especially when the underlying price is within the two breakeven points
-
Because this strategy involves selling two options, it will require a greater margin in your trading account at the time of initiation
-
A potential to lose the entire net debit amount, in case the underlying falls below the lower strike or rises above the higher strike
Strategy Suggestions
-
See that your view on the underlying is range bound
-
Avoid executing this strategy when you expect volatility to pick up. Instead this strategy works best when volatility is low/declining
-
Ensure that the distance between the lower strike and the middle strike is equal to that between the middle strike and the higher strike
-
The spread between the strikes will be a trade-off between the cost/risk of the strategy and the potential reward
-
The narrow the difference between the strikes, the smaller will be the cost/risk but so would be the reward, and vice versa
-
Because this is a range bound strategy that benefits from low volatility, ensure that you execute this strategy when the expiration is not far off, as giving too much time could cause the underlying price to move beyond either of the two breakeven points
-
Ensure there is sufficient liquidity in the underlying that is being chosen to initiate this strategy
Option Greeks for Long Put Butterfly
| Greek | Notes |
| Delta |
Delta is at or near zero at initiation. It tends to peak out above zero when the underlying price is near the lower strike and bottom out below zero when the underlying price is near the higher strike. That said, while Delta does become non-zero as the underlying price moves, it does not deviate much from zero. As such, changes in the price of the underlying do not have much impact on this strategy because of the way it is structured. |
| Gamma |
Gamma is negative and is at its lowest point at initiation. As the underlying price moves away from the middle strike and approaches either the lower or the higher strike, Gamma tends to move into positive before peaking out around these extreme strikes. |
| Vega |
Vega is negative and is at its lowest point at initiation, meaning the negative impact of a rise in volatility is the highest at the middle strike. Volatility hurts as long as the position in profitable. That said, Vega turns positive when the position becomes unprofitable, meaning a rise in volatility now starts helping the position. |
| Theta |
Theta is positive and is at its highest point at initiation, meaning time decay is most helpful around the middle strike. Time decay benefits the position as long as it is profitable. That said, Theta turns negative when the position becomes unprofitable, meaning time decay now starts hurting the position. |
Payoff of Long Put Butterfly
The above is the payoff chart of a long Put Butterfly. Notice that the payoff chart of a long Put Butterfly is the same as that of a long Call Butterfly. Observe that the trader makes a profit when the underlying price is within the two breakeven points, with maximum profit occurring when the underlying price is exactly at the middle strike. Also notice that the trader incurs a loss when the underlying price moves outside the range of the breakeven points. Maximum loss under this strategy occurs when the underlying price is either below the lower strike price or above the higher strike price.
Example of Long Put Butterfly
Let us say that Mr. ABC has looked at the chart of Hindustan Unilever and is of the opinion that the stock will not only remain very range bound in the short-term, but the volatility will also reduce going forward. Based on this, let us say that he has decided to initiate a long Put Butterfly strategy, wherein he will buy 1 OTM 2100 Put at ₹65, sell 2 ATM 2200 Puts at ₹115 each, and buy 1 ITM 2300 Put at ₹180. Let us summarize the details of the strategy below:
-
Strike price of OTM longPut = 2100
-
Strike price of ATM shortPuts = 2200
-
Strike price of ITM long Put = 2300
-
Quantity of Puts bought = 2 lots (at different strikes)
-
Quantity of Puts sold = 2 lots (at same strike)
-
LongPut premium (lower strike) = ₹65
-
Short Put premium (middle strike) = ₹115
-
LongPut premium (higher strike) = ₹180
-
Net Debit = ₹15 (65 + 180 - 2 * 115)
-
Net Debit (in value terms) = ₹4,500 (15 * 300)
-
Lower Breakeven point = 2115 (2100 + 15)
-
Upper Breakeven point = 2285 (2300 - 15)
-
Maximum reward = ₹25,500 ((2200 - 2100 - 15) * 300)
-
Maximum risk = ₹4,500
Now, let us assume a few scenarios in terms of where Hindustan Unilever would be on the expiration date and the impact this would have on the profitability of the trade.
| Underlying price at Expiration | Net Profit/Loss | Notes |
| 1800 | Loss of ₹4,500 | Payoff = [Maximum of (2100-1800,0)-65]+[2*{115-maximum of (2200-1800,0)}] + [Maximum of (2300-1800,0)-180]. As the underlying price at expiration is below the lower breakeven point, the trader will incur a loss |
| 2000 | Loss of ₹4,500 | Payoff = [Maximum of (2100-2000,0)-65]+[2*{115-maximum of (2200-2000,0)}] + [Maximum of (2300-2000,0)-180]. As the underlying price at expiration is below the lower breakeven point, the trader will incur a loss |
| 2100 | Loss of ₹4,500 | Payoff = [Maximum of (2100-2100,0)-65]+[2*{115-maximum of (2200-2100,0)}] + [Maximum of (2300-2100,0)-180]. As the underlying price at expiration is below the lower breakeven point, the trader will incur a loss |
| 2110 | Loss of ₹1,500 | Payoff = [Maximum of (2100-2110,0)-65]+[2*{115-maximum of (2200-2110,0)}] + [Maximum of (2300-2110,0)-180]. As the underlying price at expiration is below the lower breakeven point, the trader will incur a loss |
| 2115 | No profit, No loss | Payoff = [Maximum of (2100-2115,0)-65]+[2*{115-maximum of (2200-2115,0)}] + [Maximum of (2300-2115,0)-180]. As the underlying price at expiration is equal to the lower breakeven point, the trader will neither make a profit nor incur a loss |
| 2150 | Profit of ₹10,500 | Payoff = [Maximum of (2100-2150,0)-65]+[2*{115-maximum of (2200-2150,0)}] + [Maximum of (2300-2150,0)-180]. As the underlying price at expiration is between the two breakeven points, the trader will make a profit |
| 2200 | Profit of ₹25,500 | Payoff = [Maximum of (2100-2200,0)-65]+[2*{115-maximum of (2200-2200,0)}] + [Maximum of (2300-2200,0)-180]. As the underlying price at expiration is between the two breakeven points, the trader will make a profit |
| 2250 | Profit of ₹10,500 | Payoff = [Maximum of (2100-2250,0)-65]+[2*{115-maximum of (2200-2250,0)}] + [Maximum of (2300-2250,0)-180]. As the underlying price at expiration is between the two breakeven points, the trader will make a profit |
| 2285 | No profit, No loss | Payoff = [Maximum of (2100-2285,0)-65]+[2*{115-maximum of (2200-2285,0)}] + [Maximum of (2300-2285,0)-180]. As the underlying price at expiration is equal to the upper breakeven point, the trader will neither make a profit nor incur a loss |
| 2290 | Loss of ₹1,500 | Payoff = [Maximum of (2100-2290,0)-65]+[2*{115-maximum of (2200-2290,0)}] + [Maximum of (2300-2290,0)-180]. As the underlying price at expiration is above the upper breakeven point, the trader will incur a loss |
| 2300 | Loss of ₹4,500 | Payoff = [Maximum of (2100-2300,0)-65]+[2*{115-maximum of (2200-2300,0)}] + [Maximum of (2300-2300,0)-180]. As the underlying price at expiration is above the upper breakeven point, the trader will incur a loss |
| 2400 | Loss of ₹4,500 | Payoff = [Maximum of (2100-2400,0)-65]+[2*{115-maximum of (2200-2400,0)}] + [Maximum of (2300-2400,0)-180]. As the underlying price at expiration is above the upper breakeven point, the trader will incur a loss |
| 2500 | Loss of ₹4,500 | Payoff = [Maximum of (2100-2500,0)-65]+[2*{115-maximum of (2200-2500,0)}] + [Maximum of (2300-2500,0)-180]. As the underlying price at expiration is above the upper breakeven point, the trader will incur a loss |
Notice in the above table that maximum loss under this strategy is limited and occurs when HUL drops below the lower strike of 2100 or above the higher strike of 2300. On the other side, notice that maximum profit is also limited and occurs when HUL is exactly at the middle strike of 2200. Observe that the trader will be in a profitable position as long as HUL is trading between the two breakeven points of 2115 and 2285. If HUL moves out of this range, the trader must consider whether or not to hold on to the position.
SHORT PUT BUTTERFLY
| Strategy Details | |
| Strategy Type | Neutral on direction, but bullish on volatility |
| # of legs | 4 (Short 1 Lower Strike Put + Long 2 Middle Strike Puts + Short 1 Higher Strike Put) |
| Maximum Reward | Limited to the extent of net premium received |
| Maximum Risk | Middle Strike Price - Lower Strike Price - Net Premium Received |
| Lower Breakeven Price | Lower Strike Price + Net Premium Received |
| Upper Breakeven Price | Higher Strike Price - Net Premium Received |
| Payoff Calculation | Payoff of lower strike Short Put + (2 * Payoff of middle strike Long Put) + Payoff of higher strike Short Put |
Explaination of the Strategy
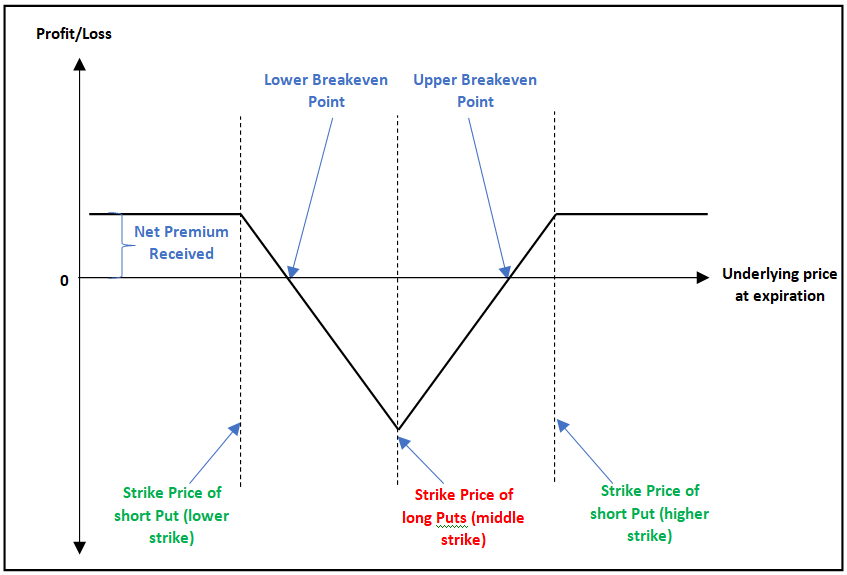
A Short Put Butterfly is a strategy that involves writing a lower strike Put, buying two middle strike Puts, and again writing a higher strike Put. The lower strike Put that is written is an OTM Put, the middle strike Puts that are bought are usually ATM Puts, and the higher strike Put that is written is an ITM Put. All these options must belong to the same underlying and must have the same expiration date. Meanwhile, the lower and the higher strike must be equidistant from the middle strike.
A Short Put Butterfly is a net credit strategy, in which the maximum profit potential is limited to the extent of net premium received. For the strategy to achieve its maximum profit potential, the underlying price will have to either fall below the lower strike price or rise above the higher strike price. For this to happen, volatility is of paramount importance. The higher the volatility, the higher the odds of the underlying price swinging in one or the other direction. Hence, it can be said that this strategy is neutral on direction but bullish on volatility.
A Short Put Butterfly has two breakeven points: lower and upper. The trader is in a profitable position as long as the underlying price is either below the lower breakeven point or above the upper breakeven point. On the flip side, if the underlying price consolidates between the two breakeven points, the trader will suffer a loss. Maximum loss under this strategy occurs when the underlying price is exactly at the middle strike. Hence, execute this strategy only when you expect the underlying price to either fall below the lower strike or rise above the higher strike.
Benefits of the Strategy
-
This is a net credit strategy, meaning there is no cash outflow at the time of initiation
-
Maximum loss under this strategy is limited
-
This strategy benefits from a surge in volatility
Drawbacks of the Strategy
-
This strategy has limited profit potential
-
The risk reward profile of initiating this strategy is highly unattractive as risk is larger than reward
-
Time decay hurts the position when the underlying price is in between the two breakeven points
Strategy Suggestions
-
Ensure that the underlying is trading within a range and you expect this range to hold
-
Within this trading range, have conviction that the underlying will either rise to or slightly above the upper end of the range or fall to or slightly below the lower end of the range
-
Execute this strategy when you expect volatility to pick up or when it is already high and is likely to remain so
-
Ensure that the distance between the lower strike and the middle strike is equal to that between the middle strike and the higher strike
-
The spread between the strikes will be a trade-off between risk and reward
-
The wider the difference between the strikes, the higher will be the reward but so would be the risk, and vice versa
-
As the risk involved in trading this strategy is higher than the reward, this strategy must preferably be executed by experienced traders only
-
Because you want the underlying price to move away from the middle strike and because you want volatility to rise, give yourself sufficient time to go right
-
Ensure there is sufficient liquidity in the underlying that is being chosen to initiate this strategy
Option Greeks for Short Put Butterfly
| Greek | Notes |
| Delta |
Delta is at or near zero at initiation. It tends to bottom out below zero when the underlying price is near the lower strike and peak out above zero when the underlying price is near the higher strike. That said, while Delta does become non-zero as the underlying price moves, it does not deviate much from zero. As such, changes in the price of the underlying do not have much impact on this strategy because of the way it is structured. |
| Gamma |
Gamma is positive and is at its highest point at initiation. As the underlying price moves away from the middle strike and approaches either the lower or the higher strike, Gamma tends to move into negative before bottoming out around these extreme strikes. |
| Vega |
Vega is positive and is at its highest point at initiation, meaning the positive impact of a rise in volatility is the greatest at the middle strike. Volatility benefits as long as the position in unprofitable. That said, Vega turns negative when the position becomes profitable, meaning a rise in volatility now starts hurting the position. |
| Theta |
Theta is negative and is at its lowest point at initiation, meaning time decay hurts the most around the middle strike. Time decay hurts the position as long as it is unprofitable. That said, Theta turns positive when the position becomes profitable, meaning time decay now starts benefiting the position. |
Payoff of Short Put Butterfly
The above is the payoff chart of a Short Put Butterfly. Observe that this strategy is profitable outside the two breakeven points. The sweetest spot is when the underlying price is either below the lower strike or above the higher strike. On the other hand, this strategy is unprofitable when the underlying price is between the two breakeven points. See that the maximum loss under this strategy is limited and occurs when the underlying price is exactly at the middle strike.
Example of Short Put Butterfly
Let us say that Mr. ABC has looked at the chart of Hindustan Unilever and is of the opinion that the stock will remain very range bound in the short-term. However, he is also of the opinion that volatility will rise going forward. Based on this, let us say that he has decided to initiate a short Put Butterfly strategy, wherein he will sell 1 OTM 2000 Put at ₹40, buy 2 ATM 2200 Puts at ₹115 each, and sell 1 ITM 2400 Put at ₹245. Let us summarize the details of the strategy below:
-
Strike price of OTM shortPut = 2000
-
Strike price of ATM longPuts = 2200
-
Strike price of ITM short Put = 2400
-
Quantity of Puts bought = 2 lots (at same strikes)
-
Quantity of Puts sold = 2 lots (at different strike)
-
ShortPut premium (lower strike) = ₹40
-
Long Put premium (middle strike) = ₹115
-
ShortPut premium (higher strike) = ₹245
-
Net Credit = ₹55 (40 + 245 - 2 * 115)
-
Net Credit (in value terms) = ₹16,500 (55 * 300)
-
Lower Breakeven point = 2055 (2000 + 55)
-
Upper Breakeven point = 2345 (2400 - 55)
-
Maximum reward = ₹16,500
-
Maximum risk = ₹43,500 ((2200 - 2000 - 55) * 300)
Now, let us assume a few scenarios in terms of where Hindustan Unilever would be on the expiration date and the impact this would have on the profitability of the trade.
| Underlying price at Expiration | Net Profit/Loss | Notes |
| 1700 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-1700,0)]+[2*{Maximum of (2200-1700,0)-115}] + [245-Maximum of (2400-1700,0)]. As the underlying price at expiration is below the lower breakeven point, the trader will make a profit |
| 1900 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-1900,0)]+[2*{Maximum of (2200-1900,0)-115}] + [245-Maximum of (2400-1900,0)]. As the underlying price at expiration is below the lower breakeven point, the trader will make a profit |
| 2000 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-2000,0)]+[2*{Maximum of (2200-2000,0)-115}] + [245-Maximum of (2400-2000,0)]. As the underlying price at expiration is below the lower breakeven point, the trader will make a profit |
| 2030 | Profit of ₹7,500 | Payoff = [40-Maximum of (2000-2030,0)]+[2*{Maximum of (2200-2030,0)-115}] + [245-Maximum of (2400-2030,0)]. As the underlying price at expiration is below the lower breakeven point, the trader will make a profit |
| 2055 | No profit, No loss | Payoff = [40-Maximum of (2000-2055,0)]+[2*{Maximum of (2200-2055,0)-115}] + [245-Maximum of (2400-2055,0)]. As the underlying price at expiration is equal to the lower breakeven point, the trader will neither profit nor incur a loss |
| 2100 | Loss of ₹13,500 | Payoff = [40-Maximum of (2000-2100,0)]+[2*{Maximum of (2200-2100,0)-115}] + [245-Maximum of (2400-2100,0)]. As the underlying price at expiration is between the two breakeven points, the trader will incur a loss |
| 2200 | Loss of ₹43,500 | Payoff = [40-Maximum of (2000-2200,0)]+[2*{Maximum of (2200-2200,0)-115}] + [245-Maximum of (2400-2200,0)]. As the underlying price at expiration is between the two breakeven points, the trader will incur a loss |
| 2300 | Loss of ₹13,500 | Payoff = [40-Maximum of (2000-2300,0)]+[2*{Maximum of (2200-2300,0)-115}] + [245-Maximum of (2400-2300,0)]. As the underlying price at expiration is between the two breakeven points, the trader will incur a loss |
| 2345 | No profit, No loss | Payoff = [40-Maximum of (2000-2345,0)]+[2*{Maximum of (2200-2345,0)-115}] + [245-Maximum of (2400-2345,0)]. As the underlying price at expiration is equal to the upper breakeven point, the trader will neither profit nor incur a loss |
| 2380 | Profit of ₹10,500 | Payoff = [40-Maximum of (2000-2380,0)]+[2*{Maximum of (2200-2380,0)-115}] + [245-Maximum of (2400-2380,0)]. As the underlying price at expiration is above the upper breakeven point, the trader will earn a profit |
| 2400 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-2400,0)]+[2*{Maximum of (2200-2400,0)-115}] + [245-Maximum of (2400-2400,0)]. As the underlying price at expiration is above the upper breakeven point, the trader will earn a profit |
| 2500 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-2500,0)]+[2*{Maximum of (2200-2500,0)-115}] + [245-Maximum of (2400-2500,0)]. As the underlying price at expiration is above the upper breakeven point, the trader will earn a profit |
| 2700 | Profit of ₹16,500 | Payoff = [40-Maximum of (2000-2700,0)]+[2*{Maximum of (2200-2700,0)-115}] + [245-Maximum of (2400-2700,0)]. As the underlying price at expiration is above the upper breakeven point, the trader will earn a profit |
Notice in the above table that maximum profit under this strategy is limited and occurs when HUL drops below the lower strike of 2000 or rises above the higher strike of 2400. On the other side, notice that maximum loss is limited and occurs when HUL is exactly at the middle strike of 2200. Observe that the trader will be in a loss-making position as long as HUL is trading between the two breakeven points of 2055 and 2345. It is when HUL moves out of this range that the trader will start making profits. Meanwhile, observe the risk to reward ratio for this strategy. With a maximum possible profit of ₹16,500 and a maximum possible loss of ₹43,500, the maximum risk turns out to be 2.5 times the maximum reward. Hence, from a risk to reward perspective, this strategy is quite unfavourable and must be executed by experienced traders only.
Next Chapter
Comments & Discussions in
FYERS Community