
In this chapter, we shall study some crucial concepts that pertain to options. These include the two elements of option price (intrinsic value and time value) and moneyness of an Option. We will also cover how to calculate the breakeven point of an option, how to calculate the profit/loss potential of an option and discuss about the option payoff charts. We will conclude this chapter by talking about the key terminologies that were covered over the course of this chapter.
Intrinsic value, Time value, and Moneyness of an Option
Now that we have understood the basics of options, it is time to move on to the other aspects of an option contract.
Elements of an Option price
As we saw earlier, an option price, which is also known as the option premium, is the price that an option buyer must pay to the option seller to acquire the right to the option. There are two elements to an option price: intrinsic value and time value. In other words,
Option price = intrinsic value + time value
Let us now understand what each of these elements mean.
Intrinsic value of an Option
Intrinsic value of an option measures the extent to which an option is In-the-Money (ITM).It can be calculated by finding the difference between the strike price of an option and the corresponding current price of the underlying. At any point in time, the intrinsic value of an option could be either positive or zero. It cannot be negative. A positive intrinsic value occurs when the holder of the option contract stands to benefit by exercising his right to the option, while a zero intrinsic value occurs when the holder of the option contract wouldn’t benefit by exercising his right to the option. For instance, if the strike price of a call option is ₹100 and the prevailing price of the corresponding underlying is ₹120, the call option would have a positive intrinsic value because the call buyer can benefit by exercising his right to buy the underlying at ₹100 and then sell it in the market for ₹120, thereby profiting ₹20, which is nothing but the intrinsic value. On the other hand, if the strike price of a put option is ₹100 and the prevailing price of the corresponding underlying is ₹120, the put option would have no intrinsic value because the put buyer wouldn’t benefit by exercising his right to the option and would rather be better off by selling the underlying at the prevailing market price.
Intrinsic value of a call: A call option will have a positive intrinsic value when its strike price is below the current market price. On the other hand, a call option will have no intrinsic value when its strike price is equal to or above the current market price
Intrinsic value of a call = current price - strike price
If the above value is positive, it means the call option has an intrinsic value. If it is zero or negative, it means the call option has no intrinsic value.
Intrinsic value of a put: A put option will have a positive intrinsic value when its strike price is above the current market price. On the other hand, a put option will have no intrinsic value when its strike price is equal to or below the current market price.
Intrinsic value of a put = strike price - current price
If the above value is positive, it means the put option has an intrinsic value. If it is zero or negative, it means the put option has no intrinsic value.
Keep in mind that intrinsic value cannot be negative. As such, if the intrinsic value of a call or a put turns out to be negative, then it is construed as zero.
Time value of an Option
While intrinsic value is the tangible portion of the option price, time value is the intangible portion of the option price. It is also known as the extrinsic value of an option. The time value of an option is determined not by the price of the underlying, but rather by the time to expiration of the option. The greater the time to expiration, the greater would be the time value of an option, and vice versa. As the time to expiration reduces, so does the time value. At expiration, the time value of an option would be zero. The reason why the time value gradually erodes is because of a concept of time decay. The greater the time to expiration, the higher would be the probability of the option price moving in favour of the option buyer, and vice versa. As the time to expiration reduces, so does the probability of an option price moving in favour of the option buyer. Time value of an option could also be thought of as a part of the option price that a writer receives for taking the time risk.
The price of an option would be readily available on the options screen, while the intrinsic value of an option can be calculated by finding the difference between the strike price and the current market price of the underlying. What is left over in the option price equation that was mentioned earlier is the time value of the option, which can be calculated as:
Time value = option price - intrinsic value
So, for instance, let us assume that the strike price of a call option is ₹100, the current price of the underlying is ₹112, and the option price is ₹15. The intrinsic value of this call option is ₹12 (₹112 current price - ₹100 strike price). Now that we know the option price (₹15) and the intrinsic value (₹12), the time value of the call option can be calculated as ₹3 (₹15 option price - ₹12 intrinsic value). This ₹3 is the excess premium that a seller would make for taking the time risk.
On the other hand, what if the strike price of a call option is ₹100, the current price of the underlying is ₹90, and the option price is ₹5? In this case, the intrinsic value would be zero (because negative intrinsic values are considered as zero). As such, the entire option price of ₹5 would reflect the time value of the option. In other words, this option would have only time value and no intrinsic value. An important thing to make out of this is that for options whose intrinsic value is zero, the entire option price reflects the time value of the option.
Moneyness of an Option
Now that we have understood what intrinsic value and time value of an option is, it is time to talk about another crucial section that is called moneyness of an option. At any point in time, an option could be in any one of the three forms:
In-the-Money (ITM)
At-the-Money (ATM)
Out-of-the-Money (OTM)
Let us split each of the above into two groups: one for a call option and the other for a put option.
- In-the-Money (ITM) Call option: A call option is said to be ITM when the strike price of that option is below the corresponding current market price of the underlying. In other words, if (Market price - Strike price) > 0, a call option is said to be ITM. Notice that an ITM call option will have a positive intrinsic value.
- At-the-Money (ATM) Call option: A call option is said to be ATM when the strike price of that option is equal to the corresponding current market price of the underlying. In other words, if (Market price - Strike price) = 0, a call option is said to be ATM.Notice that an ATM call option will have no intrinsic value.
- Out-of-the-Money (OTM) Call option: A call option is said to be OTM when the strike price of that option is above the corresponding current market price of the underlying. In other words, if (Market price - Strike price) < 0, a call option is said to be OTM. Notice that an OTM call option will have no intrinsic value.
- In-the-Money (ITM) Put option: A put option is said to be ITM when the strike price of that option is above the corresponding current market price of the underlying. In other words, if (Strike price - Market price) > 0, a put option is said to be ITM. Notice that an ITM put option will have a positive intrinsic value.
- At-the-Money (ATM) Put option: A Put option is said to be ATM when the strike price of that option is equal to the corresponding current market price of the underlying. In other words, if (Strike price - Market price) = 0, a put option is said to be ATM.Notice that an ATM put option will have no intrinsic value.
- Out-of-the-Money (OTM) Put option: A put option is said to be OTM when the strike price of that option is below the corresponding current market price of the underlying. In other words, if (Strike price - Market price) < 0, a put option is said to be OTM. Notice that an OTM put option will have no intrinsic value.
Let us now talk each of intrinsic value, time value, and option moneyness from a practical standpoint, using the two tables below:
| Option Type | Strike Price | Market Price | Option Premium | ITM / ATM / OTM? | Intrinsic Value | Time Value |
| Call | 80 | 100 | 28 | ITM | 20 | 8 |
| Call | 90 | 100 | 17 | ITM | 10 | 7 |
| Call | 100 | 100 | 6 | ATM | 0 | 6 |
| Call | 110 | 100 | 4 | OTM | 0 | 4 |
| Call | 120 | 100 | 3 | OTM | 0 | 3 |
| Option Type | Strike Price | Market Price | Option Premium | ITM/ATM/OTM? | Intrinsic Value | Time Value |
| Put | 120 | 100 | 30 | ITM | 20 | 10 |
| Put | 110 | 100 | 18 | ITM | 10 | 8 |
| Put | 100 | 100 | 7 | ATM | 0 | 7 |
| Put | 90 | 100 | 5 | OTM | 0 | 5 |
| Put | 80 | 100 | 2 | OTM | 0 | 2 |
An easy way to remember each of the concept learned about intrinsic value, time value, and option moneyness is to ask yourself a question: If I were the option buyer, would I exercise this option at the prevailing market price of the underlying? You would do so only if you would make money by exercising the option. For instance, consider the call option table above. Would you exercise your right to buy the asset at ₹80 or₹90 when the prevailing market price of the underlying is ₹100? You certainly would as you would be able to buy the underlying at a price that is below the current market price of the underlying. On the other hand, would you exercise your right to buy the asset at ₹110 or ₹120 when the prevailing market price of the underlying is ₹100? You certainly wouldn’t as the underlying is available for a lower price in the market, hence you would be better off by buying the underlying from the market itself. Now consider the put option table above. Would you exercise your right to sell the asset at ₹120 or ₹110 when the prevailing market price of the underlying is ₹100? You certainly would as you would be able to sell the underlying at a price that is above the current market price of the underlying. On the other hand, would you exercise your right to sell the asset at ₹90 or ₹80 when the prevailing market price of the underlying is ₹100? You certainly wouldn’t as the underlying is available for a higher price in the market, hence you would be better off by selling directly in the market itself.
Calculating Breakeven point, Profit/Loss on an Option contract
Now,we shall focus on two important concepts: One is how to calculate the breakeven point of call and put options, and the other is how to calculate the profit/loss on an option position.
First, let us understand what breakeven point is. Breakeven point, as the name suggests, is the point of no profit, no loss. It is the point where an option trader is neutral on the profit front, i.e. he is neither making money nor losing money. Now, let us see how to calculate breakeven point for calls and puts. First, let us see the equation of calculating breakeven point, and then we shall explain each of them.
Breakeven point of a long call: Strike price + option premium paid
Breakeven point of a short call: Strike price + option premium received
Breakeven point of a long put: Strike price – option premium paid
Breakeven point of a short put: Strike price – option premium received
In case of a call option, the point of no profit, no loss is the strike price plus the option premium. At this point, the buyer of a call and the seller of a call would be at breakeven. When price moves away from this point, the buyer and the seller will start making profits or losses. If the price moves to the right of the breakeven point, the buyer of a call will start making profits and the seller of a call will start incurring losses. On the other hand, if the price moves to the left of the breakeven point, the buyer of a call will start incurring losses and the seller of a call will start making profits, both of which would be limited to the extent of the premium that the buyer has paid to the seller.
Meanwhile, in case of a put option, the point of no profit, no loss is the strike price less the option premium. At this point, the buyer of a put and the seller of a put would be at breakeven. When price moves away from this point, the buyer and the seller will start making profits or losses. If the price moves to the left of the breakeven point, the buyer of a put will start making profits and the seller of a put will start incurring losses. On the other hand, if the price moves to the right of the breakeven point, the buyer of a put will start incurring losses and the seller of a put will start making profits, both of which would be limited to the extent of the premium that the buyer has paid to the seller.
Now that we understand how to calculate the breakeven point, let us see how to calculate the profit/loss potential of an option trade.
Profit/loss for the buyer of a call option
The profit/loss for the buyer of a call option can be calculated as: Underlying price – Breakeven price. If this value is positive, it means the buyer has made a gain. If it is negative, it means the buyer has incurred a loss. Keep in mind that the maximum loss cannot exceed the premium that the buyer has paid. For instance, if underlying price – breakeven price is coming as -30 while the option premium is 10, then the loss in this case to the buyer is 10 and not 30. Always remember that the loss to the buyer of an option cannot exceed the premium that he has paid.
Profit/loss for the seller of a call option
The profit/loss for the seller of a call option can be calculated as: Breakeven price – Underlying price. If this value is positive, it means the seller has made a gain. If it is negative, it means the seller has incurred a loss. Keep in mind that the maximum gain cannot exceed the premium that the seller has received. For instance, if breakeven price – underlying price is coming as 50 while the option premium is 15, then the gain in this case to the seller is 15 and not 50. Always remember that the gain to the seller of an option cannot exceed the premium that he has received.
Profit/loss for the buyer of a put option
The profit/loss for the buyer of a put option can be calculated as: Breakeven price – Underlying price. If this value is positive, it means the buyer has made a gain. If it is negative, it means the buyer has incurred a loss. Keep in mind that the maximum loss cannot exceed the premium that the buyer has paid.
Profit/loss for the seller of a put option
The profit/loss for the seller of a put option can be calculated as: Underlying price – Breakeven price. If this value is positive, it means the seller has made a gain. If it is negative, it means the seller has incurred a loss. Keep in mind that the maximum gain cannot exceed the premium that the seller has received.
Payoff Charts
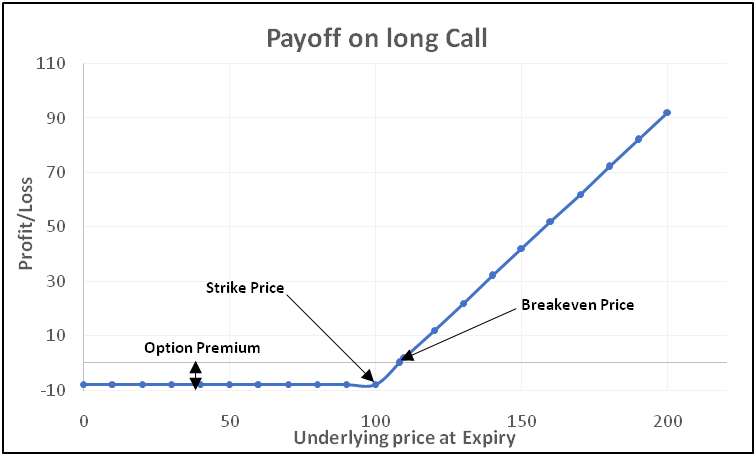
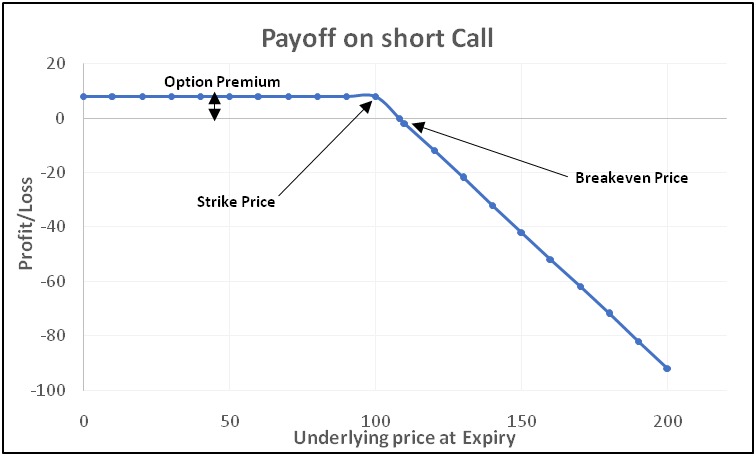
The concepts that we studied in the previous section will become easier to understand using the payoff charts, which are presented below.For each of the options payoff chart below, we shall assume a strike price of ₹100 and an option premium of ₹8.
The chart above is the payoff chart of a long call option. The breakeven point for the call is ₹108 (₹100 strike price + ₹8 option premium). Notice in the chart that as long as the underlying price is at or below ₹100, the buyer’s loss would be limited to the extent of the premium that he has paid upfront. As the price starts rising above the strike price and up to the breakeven price, the buyer’s loss will start reducing. The moment the price crosses the breakeven price, the buyer will start making gains. Observe that the buyer’s gain is potentially unlimited. The higher the price rises above the breakeven point, the higher would be the buyer’s gain.
The chart above is the payoff chart of a short call option. The breakeven point for the call is ₹108 (₹100 strike price + ₹8 option premium). Notice in the chart that as long as the underlying price is at or below ₹100, the seller would make a gain, which would be fixed to the extent of the option premium that he has received upfront, irrespective of how low the underlying price is below the strike price. As the price starts rising above the strike price and up to the breakeven price, the seller’s gain will start reducing. The moment the price crosses the breakeven price, the seller will start incurring losses. Observe that the seller’s loss is potentially unlimited. The higher the price rises above the breakeven point, the higher would be the seller’s loss.
The chart above is the payoff chart of a long put option. The breakeven point for the put is ₹92 (₹100 strike price - ₹8 option premium). Notice in the chart that as long as the underlying price is at or above ₹100, the buyer’s loss would be limited to the extent of the premium that he has paid upfront. As the price starts falling below the strike price and up to the breakeven price, the buyer’s loss will start reducing. The moment the price moves below the breakeven point, the buyer will start making gains. Observe that the buyer’s gain is potentially unlimited (until the underlying price drops to zero, of course). The lower the price drops below the breakeven point, the higher would be the buyer’s gain.
The chart above is the payoff chart of a shortput option. The breakeven point for the put is ₹92 (₹100 strike price - ₹8 option premium). Notice in the chart that as long as the underlying price is at or above ₹100, the seller would make a gain, which would be fixed to the extent of the option premium that he has received upfront, irrespective of how high the underlying price is above the strike price. As the price starts fallingbelow the strike price and up to the breakeven price, the seller’s gain will start reducing. The moment the price movesbelow the breakeven point, the seller will start incurring losses. Observe that the seller’s loss is potentially unlimited (until of course the price drops to zero). The lower the price drops below the breakeven point, the higher would be the seller’s loss.
Concluding with key Options Terminology
We shall nowconclude by discussing about the terminologies that we have learned in this chapter. Again, the concepts that we have learned in this chapterare extremely important and would form the basic blocks of things that we are going to discuss in the forthcoming chapters. Hence, it is crucial to have a very strong understanding of these terminologies. We strongly suggestthe reader to spend quality time on understanding theseterminologies, before moving ahead to the next chapter.
-
Option price:This is the price that an option buyer must pay to an option seller for acquiring the right to the option contract. It comprises of two components – time value and intrinsic value. An option price is also known as option premium.
Option price = Intrinsic value + Time value
-
In-the-money (ITM) option: A call option is said to be ITM if the current price of the underlying security is above the strike price, while a put option is said to be ITM is the current price of the underlying is below the strike price.
-
At-the-money (ATM) option: A call/put option is said to be ATM if the current price of the underlying security is equal to the strike price.
-
Out-the-money (OTM) option: A call option is said to be OTM if the current price of the underlying security is below the strike price, while a put option is said to be OTM if the current price of the underlying security is above the strike price.
-
Intrinsic value of an option:Intrinsic value refers to the amount an option is ITM. If an option is OTM, its intrinsic value is zero. For a call/put option that is ITM, intrinsic value is calculated as the absolute value of the difference between the current price of the underlying and the strike price.
-
Time value of an option:Time value is the difference between the option price and the intrinsic value. If an option is OTM, option price is equal to time value (as intrinsic value is zero). The greater the time to expiration, the higher will be the time value, and vice versa.
-
Breakeven price: This is the price at which the buyer of an option will be at breakeven, i.e. neither making a profit nor a loss. For a call option, the breakeven price is equal to strike price plus the premium paid by the buyer; while for a put option, the breakeven price is equal to strike price minus the premium paid by the buyer.
Next Chapter
Comments & Discussions in
FYERS Community


RAKESH commented on February 19th, 2020 at 9:45 PM
Simplify way to expres content , excellent Thx
Shriram commented on February 20th, 2020 at 11:03 AM
Thank you Rakesh!
Subhash Chandra Ghosh. commented on March 6th, 2020 at 10:22 PM
Unbelievable, awesome, all the adjectives in English grammar !!!